The Geometry Program addresses two of the most important aspects of mathematics instruction.
First, the inquiry-based video format contributes to the engaging of students more personally in the concept development process. Through the frequent use of the pause button, you, as the instructor, can virtually require interaction and dialogue on the part of your student. As well, students who work on their own, can “simulate” having an instructor present by pausing the lesson every time a question is asked, and trying to answer it correctly before continuing. Of course, the student may answer incorrectly, but the narrator will be sure to give the right answer when the play button is pressed to resume the lesson. Right or wrong, however, the student is regularly engaging in analytical and critical thinking, and that is a healthy exercise, in and of itself.
Second, each incremental concept is explored in detail, using no shortcuts, tricks, rules, or formulas, and no step in the process is ignored. As such, the logic and the continuity of the development assure students that they understand completely. Subsequently, learning is more efficient, and all of the required concepts (topics) of the subject can be covered with mastery.
The program covers The Structure of Geometry, Essential Elements (Fundamental Terms & Fundamental Theorems), and Simple Closed Plane Curves (Triangles, Other Polygons & Circles).
Materials in the complete course include:
Video Lessons – Each of the 5-10 minute lessons explore Geometry concepts in a detailed logical order. Because no shortcuts or tricks are used, the methods are easy to follow and promote clear understanding.
Course Notes – These notes allow students to review the logical development of a concept. Each page chronologically follows the video lesson, repeating exactly what was shown on the screen.
Student WorkText – These pages review the concept developed in each lesson. More examples are given and exercises are provided for students. The explanations are virtually free of complicated language, making it easy for students to follow the logic of each concept.
Solutions Manuals – These manuals provide detailed, step-by-step solutions for every problem in the student WorkText. This resource is a powerful tool when used by students to complete an error-analysis of their work, and to check their thought processes.
Progress Tests – These tests, with the answer keys included, are designed to have students demonstrate understanding, lesson-by-lesson, and unit-by-unit. There are two versions of each test, allowing for retesting or review, to make sure students have mastered concepts.
CLAIMED CREDITS:
With the completion of Geometry: A Complete Course, the student can claim credits for Geometry (1).
If a student previously completed Algebra: A Complete Course, the student can claim credits for all of the following:
- Algebra I (1)
- Algebra II (1)
GEOMETRY PROGRAM OVERVIEW:
The VideoText Interactive Geometry program addresses two of the most important aspects of mathematics instruction. First, the inquiry-based video format contributes to the engaging of students more personally in the concept development process. Through the frequent use of the pause button, you, as the instructor, can virtually require interaction and dialogue on the part of your student. As well, students who work on their own, can “simulate” having an instructor present by pausing the lesson every time a question is asked, and trying to answer it correctly before continuing. Of course, the student may answer incorrectly, but the narrator will be sure to give the right answer when the play button is pressed to resume the lesson. Right or wrong, however, the student is regularly engaging in analytical and critical thinking, and that is a healthy exercise, in and of itself.
Second, each incremental concept is explored in detail, using no shortcuts, tricks, rules, or formulas, and no step in the process is ignored. As such, the logic and the continuity of the development assure students that they understand completely. Subsequently, learning is more efficient, and all of the required concepts (topics) of the subject can be covered with mastery. Of course, the benefits of these efforts can be seen even more clearly in a description of a typical session, as follows:
After a brief 2 or 3 sentence introduction of the concept to be considered, usually by examining the description, and the objective given at the beginning of the video lesson, you and your student can begin. You should pause the lesson frequently, usually every 15-20 seconds (or more often if appropriate), to engage your student in discussion. This means that, for a 5-10 minute VideoText lesson, it may take 10-15 minutes to finish developing the concept. Dialogue is a cornerstone. In addition, during this time, your student should probably not be allowed to take notes. Students should not have their attention divided, or they risk missing important links. Neither should you be dividing your attention, by looking at notes, or writing on a pad, or an overhead projector. Everyone should be concentrating on concept development and understanding. Please understand that a student who is accustomed to working alone, or can be motivated to study independently, has, with the VideoText, a powerful resource to explore and master mathematical concepts by simulating the dialogue normally encountered with a “live” instructor. And, because of the extensive detail of the explanations, along with the computer generated graphics, and animation, students are never shortchanged when it comes to the insight necessary to fully comprehend.
Once the concept is developed, and the VideoText lesson is completed, you can then employ the Course Notes to review, reinforce, or to check on your student’s comprehension. These Course Notes are replications of the essential content that was viewed in the VideoText lesson, illustrating the same terms, diagrams, problems, numbers, and logical sequences. In fact, at this time, if your student needs a little more help, he or she can use the Course Notes while viewing the lesson again, using them as a guide, to re-examine the concept. The key here is that students concentrate on understanding first, and take care of documentation later.
Please understand that the goal of VideoText videos are not to necessarily replace an “instructor”, but rather to help aid in prompting students to actively engage in the instruction process. The video should never tell your students anything that hasn’t been considered or discussed (while the lesson is paused), and it should never answer questions that have not already been considered and resolved. As such, it becomes a “new breed” of chalkboard or overhead projector, whereby you, as the teacher, or your student working alone, can “write”, simply by pressing the “play” button. This is a critical point to be understood, and should serve to help you examine all of the materials and strategies from the proper perspective.
Next, your student can begin to do some work independently, either by your personal introduction of additional examples from the WorkText, or by the student immediately going to the WorkText on his or her own. The primary feature of the WorkText, beside providing problem banks with which students can work on mastery, is that objectives are restated, important terms are reviewed, and additional examples are considered, in noticeable detail, taking students, once again, through the logic of the concept development process. The premise here is simple. When students work with an instructor, whether doing exercises on their own, or working through them with other students, they are usually concentrating more on “how to do” the problems. Then, when they leave the instructor, they simply don’t take the discussion of the concept with them. The goal of this program is to provide a resource which will help students “re-live” the concept development on their own, whether for review, or for additional help. That is the focus of the Student WorkText.
Having completed the exercises for the lesson being considered, your student is now ready to use the detail in the Solutions Manual to check work and engage in error analysis. Again, it is essential to a student’s understanding that he or she find mistakes, correct them, and be required to give some explanation, either verbal or in writing, to you as the instructor. In fact, at this stage, you might even consider grading your student only on the completion of the work, not on its accuracy. Remember, this is the first time the student has tried to demonstrate understanding of a concept, and he or she may still need some fine-tuning. So, because this is part of the initial learning process, the focus should be on a careful analysis of the logic behind the work, not just the answers.
Finally, it is time to assess your student’s mastery of the concept behind the work. Just be sure you are not testing on the same day the exercises were completed. Short-term memory can trick you into thinking that you “have it”, when, in fact, you are just remembering what you did moments before. A more accurate evaluation can be made on the next day, before moving on to the next lesson. Further, the quizzes and tests in the program often utilize open-response questions which will require your student to state, in writing, his or her understanding of the concept. This often reveals much more about a student’s understanding than just checking to see if an answer on a test is correct. Remember too, that there are two versions of every quiz and test, allowing you to retest, if necessary, in order to make sure that your student has mastered the concept.
Of course, just as with the WorkText, there are detailed solutions for all of the quiz and test problems, in the Instructor’s Guide. Again, your student should be required to analyze problems that were missed, and explain why the problem should have been done differently. It is simply a fact that one of the most powerful and effective teaching tools you can employ, is to ask your students to “articulate” to you what their thinking was, as they worked toward a given answer.
As you can see, the highly interactive quality of this program, affords students a much greater opportunity than usual to grow mathematically, at a personal level, and develop confidence in their ability. That can have a tremendous impact on a student’s future pursuits, especially in an age where applications of mathematics are so important.
GEOMETRY SCOPE AND SEQUENCE RATIONAL:
There are two basic premises which drive concept development in Geometry, and these two essentials shape the logical scope and sequence of geometric content.
First, it is generally understood that Geometry is the study of spatial relations. In the same way that Algebra is the study of numerical relations (equations and inequalities), and Calculus is concerned primarily with rates of change, Geometry is a comprehensive exploration of “shapes” (as sets of points), the measurements associated with those shapes, and the relationships that can be established between those shapes. As such, no treatment of Geometry should ever investigate those relationships only individually, or in isolation. This is especially noticeable with traditional textbooks, which generally use a format which addresses them in different “chapters”. In the VideoText Interactive Geometry course, concepts are discussed from a “Unit” perspective, pursuing and connecting, in an exhaustive way, all of the outcomes associated with various possibilities for a specific relationship. Of course, as much as is possible, students need to “see” those relationships, and experience the “motion”, or “transformation”, necessary to clearly illustrate the concept. It really is impossible to put a value on the benefits of visualization, in life in general, and in Geometry in particular. So, in the VideoText Interactive Geometry program, computer-generated graphics are used extensively, along with animation and color-sequencing, in order that students can actually see the relationships develop.
The second premise is that geometric concepts should be studied utilizing all of the power and conviction that both inductive and deductive reasoning can bring to the table. In other words, it is always desirable, and helpful, for students to “experiment”, inductively, with a geometric relationship, in an effort to come to some general conclusion. Once that general conclusion has been arrived at, however, it is even more convincing if the student is able to “prove”, deductively, that the conclusion absolutely must follow, logically, from the given information. No, formal proof is not often asked for in everyday life. On the other hand, the exercise of developing that kind of thinking is invaluable, not only in some specific job-related activities, but, more generally, in the daily problem-solving situations that confront us. The VideoText Interactive Geometry program is formatted in such a way that formal proof is a cornerstone.
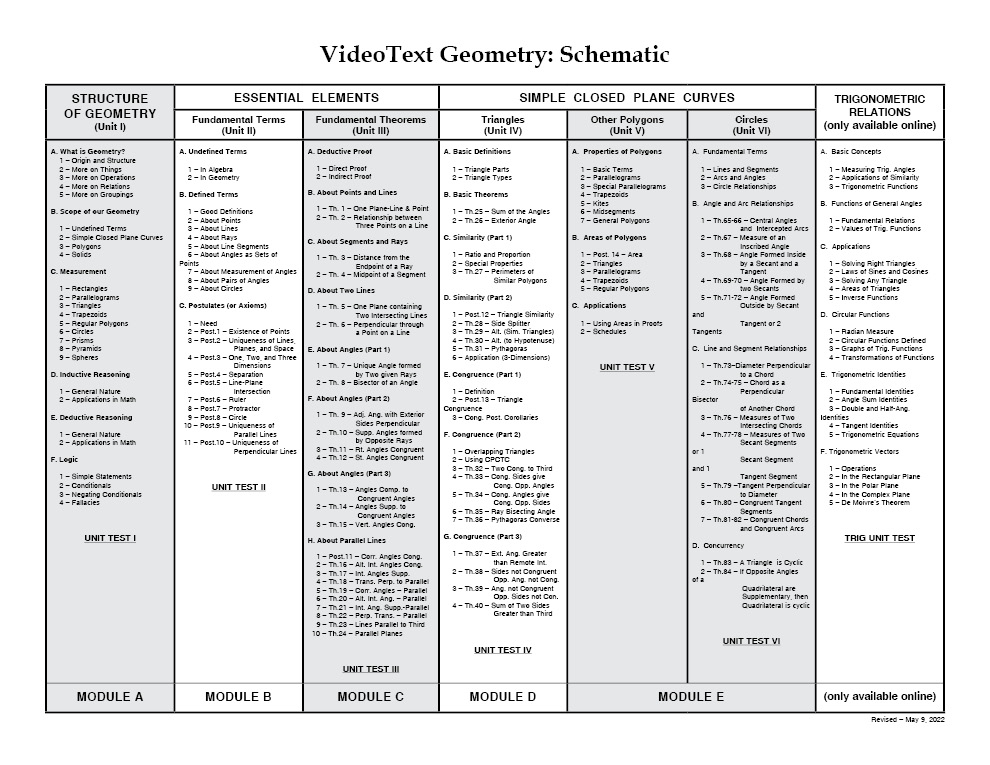
Unit I, then, focuses on a complete preparation for students to begin a formal study of Geometry by “re-teaching” of all of the basic geometric concepts for which students have simply memorized the appropriate term, definition, or formula. That means we must re-establish that Mathematics in general, and Geometry in particular, is a language, with parts of speech and sentence structure. We must develop, in detail, the concepts associated with building geometric shapes. We must investigate, again in detail, the concepts dealing with the measurement of those shapes. Finally, we must thoroughly develop the principles of inductive and deductive reasoning, giving significant attention to the dynamics of mathematical deductive logic, which are the building blocks that students will use to construct formal proofs.
In Unit II, we begin the actual study of “Plane Geometry” by developing all of the necessary terms, definitions, and assumptions we will be using as a basis for studying geometric relationships. In other words, we draw on the analogy that studying any area of Mathematics is like “playing a game”. We must first determine which basic elements will be “undefined” in our Geometry, or accepted without definition. We must then determine which basic elements can be formally defined, using those undefined terms. Finally, we must build a list of “postulates”, or conditional assumptions which will serve as the “rules of the game”, guiding us through the investigation of relationships, in our Geometry. It is important to note, at this point, that every Plane Geometry study will, in certain ways, be unique to the philosophy of the instructor, depending on the acceptance of these fundamental terms. In other words, while the prevailing context will always be that of classical Euclidean Geometry, the lists of definitions and postulates may differ from person to person. The key, however, is that each study will rely on its own particular list of Essential Elements to prove the rest of the relationships to be investigated.
So, in Unit III, we use the Fundamental Terms developed in Unit II, to prove Fundamental Theorems related to points, lines, rays, segments, and angles. These theorems will be foundational to the study of Simple Closed Plane Curves, which are the primary backdrop of all studies of Plane Geometry.
At this point, since we have put in place the “rules of the game”, we can begin, and, for all practical purposes, complete, a methodic investigation of the geometric relationships associated with Triangles (Unit IV), Other Polygons (Unit V), and Circles (Unit VI).
In the Trigonometry Unit (available online only), we will examine, in significant detail, the relationships between the various components of triangles. Included are the basic relationships of sine, cosine, and tangent, as well as applications involving the Pythagorean Theorem, the Laws of Sines and Cosines, and several other ambiguous cases.
Please understand that the organizational argument presented here is not meant to stifle the creativity of the instructor. Neither should it prohibit the instructor from utilizing a modular approach to concept development. It does, however, serve to remedy the fragmented, isolated topic, “chapter” approach to a subject which has been traditionally presented to us in “textbooks”, without that element of developmental continuity. To that end, it speaks loudly to the curricular issues which all instructors face, and the attitudinal issues students deal with when they are presented with a new and different Mathematics course.
CLICK HERE To Download the Geometry Scope and Sequence Rational
GEOMETRY SCHEMATIC: